
#1 Best Selling IGNOU Assignments in All Available in Market
Bought By: 3962 Students
Rating: 3.9
Get IGNOU MTE-14 Assignments Soft Copy ready for Download in PDF for (January 2023 - December 2023) in English Language.
Are you looking to download a PDF soft copy of the Solved Assignment MTE-14 - Mathematical Modelling? Then GullyBaba is the right place for you. We have the Assignment available in English language.
This particular Assignment references the syllabus chosen for the subject of Mathematics, for the January 2023 - December 2023 session. The code for the assignment is MTE-14 and it is often used by students who are enrolled in the B.Sc. Degree.
Once students have paid for the Assignment, they can Instantly Download to their PC, Laptop or Mobile Devices in soft copy as a PDF format. After studying the contents of this Assignment, students will have a better grasp of the subject and will be able to prepare for their upcoming tests.
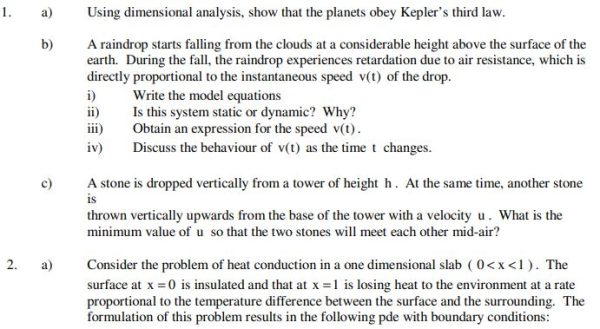

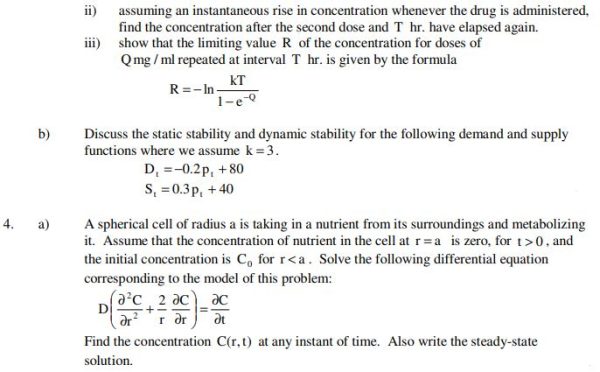

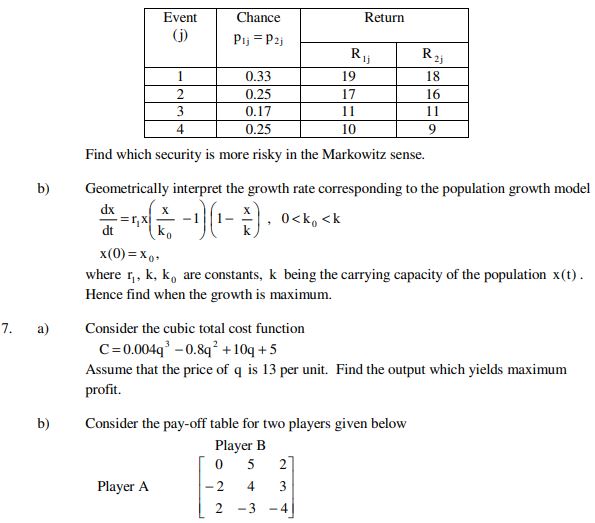
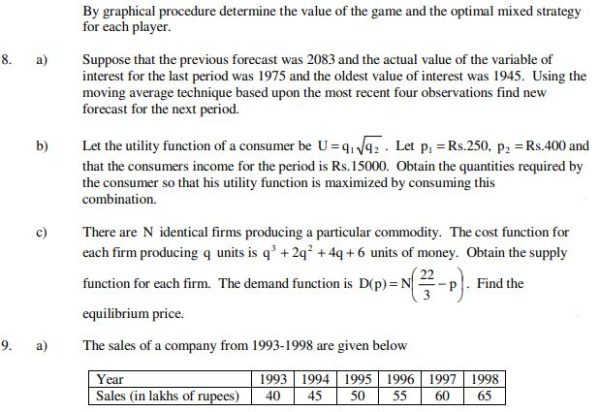


The IGNOU open learning format requires students to submit study Assignments. Here is the final end date of the submission of this particular assignment according to the university calendar.
Here are the PDF files that you can Download for this Assignment. You can pick the language of your choice and see other relevant information such as the Session, File Size and Format.
In this section you can find other relevant information related to the Assignment you are looking at. It will give you an idea of what to expect when downloading a PDF soft copy from GullyBaba.
In addition to this Assignment, there are also other Assignments related to the B.Sc. Mathematics you are preparing for. Here we have listed other Assignments that were bought along with this one.